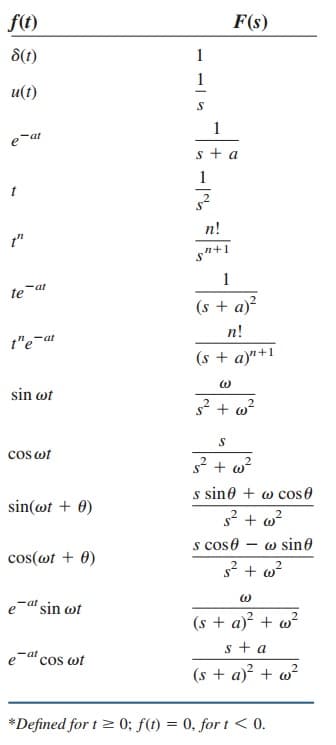
Inverse Laplace Transform Table

Co cos s sin O 23. We make use of the Laplace transform table only to get the inverse.

Solved Differential Equation Find The Indicated Laplace Chegg Com

We need to be well-versed with the Laplace transform to perform the inverse Laplace transform.

. The inverse of a complex function Fs to generate a real-valued function ft is an inverse Laplace transformation of the function. Inverse Laplace Transform Example with Partial Fractions Decomposition. Fs s 19 s 2 3s 10 Solution.

Notes Quick Nav Download. Obviously an inverse Laplace transform is the opposite process in which starting from a function in the frequency domain Fs we obtain its corresponding function in the time domain ft. How to use Laplace Transform Table for simple Inverse Laplace Transform.

Choose the pattern that matches that in the Table and perform your calculation. Because the Laplace transform is a linear operator. Usually when we compute a Laplace transform we start with a time-domain function ft and end up with a frequency-domain function Fs.

INVERSE LAPLACE TRANSFORMS In this appendix we provide additional unilateral Laplace transform pairs in Table B1 and B2 giving the s-domain expression first. The inverse Laplace transform allows us to reverse the process of Laplace transformation. For example When it comes to the inverse Laplace transform I can only find the tables to remember in a book.

This list is not a complete listing of Laplace transforms and only contains some of the more commonly used Laplace transforms and formulas. 20-28 INVERSE LAPLACE TRANSFORM Find the inverse transform indicating the method used and showing the details. Use the inverse Laplace to find ft.

We show several examples and expla. S 29-37 ODEs AND SYSTEMS LAPLACE TRANSFORMS Find the transform indicating the method used and showing Solve by the. This differential equations video explains some basic inverse Laplace transform examples using a Laplace transform chart.

6s 1 25. If a unique function is continuous on 0 to limit and also has the property of Laplace Transform. Be careful when using.

The inverse Laplace transform is exactly as named the inverse of a normal Laplace transform. Means that any table of Laplace transforms such as table 241 on page 484 is also a table of inverse Laplace transforms. Cosht et et 2 sinht etet 2 cosh.

We again work a variety of examples illustrating how to use the table of Laplace transforms to do this as well as some of the manipulation of the given Laplace transform that is needed in order to use the table. However if we go back to the Laplace transform we can always do. Simplify Fs so that we can identify the inverse Laplace transform formula.

Inverse Laplace Transform Table. Always apply the theory on which the Inverse Laplace transform is based for a quick answer. Equation for Example 2 b-1.

We check that the given Fs is of which form in the right-hand side of the Laplace transform table and the result will be the left-hand side of the identity with which it matches. T e t e t 2. Recall the definition of hyperbolic functions.

Thus by looking at Part 1 and table 1 formula F. T e t e t 2 sinh. 3s 4 27.

The following table provides Laplace transforms for many common functions of a single variable. Instead of reading off the Fs for each f t found read off the f t for each Fs. TABLE B2 Inverse Laplace Transforms.

This function is therefore an exponentially restricted real function. Identifying the general solution of the inverse Laplace transform from the table. S2 6252 10 -2s2 21.

To determine the transform with out a table. Because of this calculating the inverse Laplace transform can be used to check ones work after calculating a normal Laplace transform. The easiest way to find the inverse Laplace transform of functions is by having a table of transformations ready.

Sinusoidal and Hyperbolic LaplaceTransformXs Time-Domainxt ROC. Identify a function on the Laplace transform table that has a similar denominator. These tables are useful.

Is there an inverse analog. Free Inverse Laplace Transform calculator - Find the inverse Laplace transforms of functions step-by-step. As you may have already noticed we take inverse transforms of functions of s.

In this article well show you how an inverse Laplace transform operator works and the essential properties defining this relationship. For definitions and explanations see the Explanatory Notes at the end of the table. With a Laplace transform we can remember common set ups.

An inverse Laplace transform can only be performed on a function F s such that L f t F s exists. Compare the style of the function for which the Inverse Laplace is being computed with the stylization in the Laplace Transform Table. By the residue theorem the inverse Laplace transform depends only upon the poles and their residues.

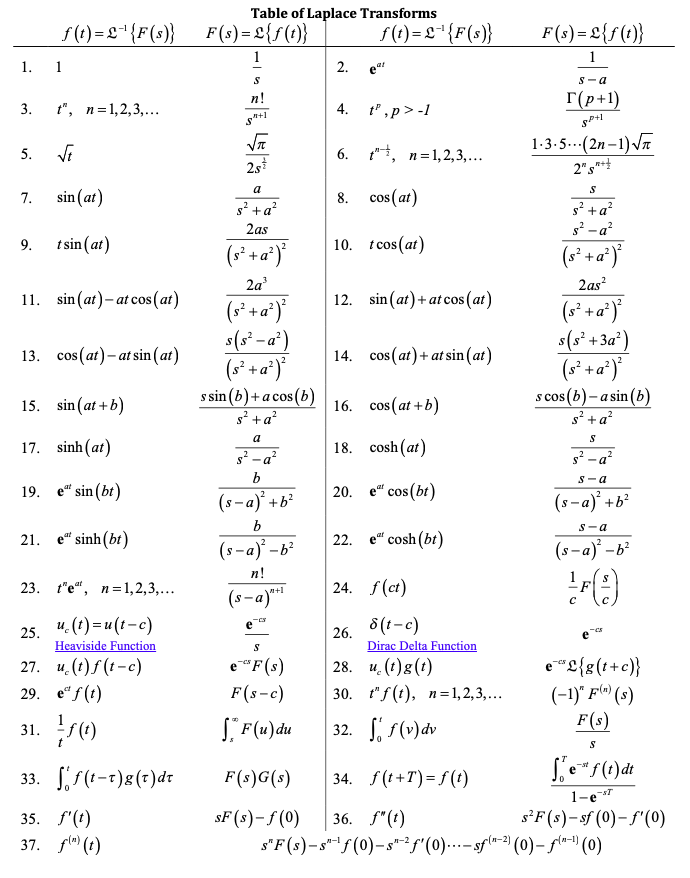
Solved You May Use The Attached Table Of Laplace Transforms Chegg Com

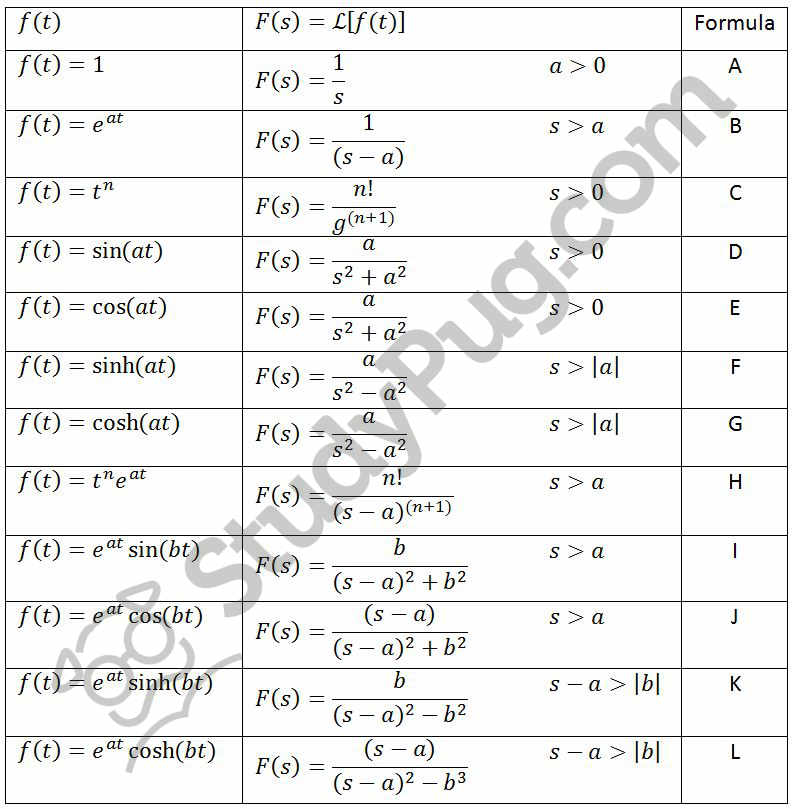
Inverse Laplace Transforms Studypug

Ordinary Differential Equations Finding Inverse Laplace Transform Mathematics Stack Exchange

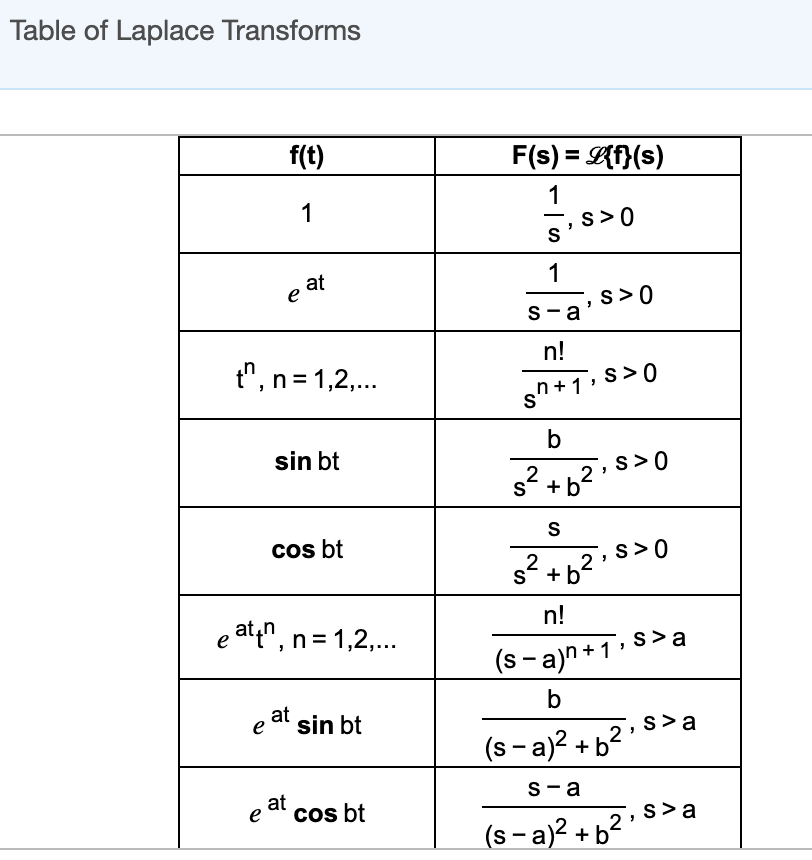
Solved 7 4 8 Determine The Inverse Laplace Transform Of The Chegg Com

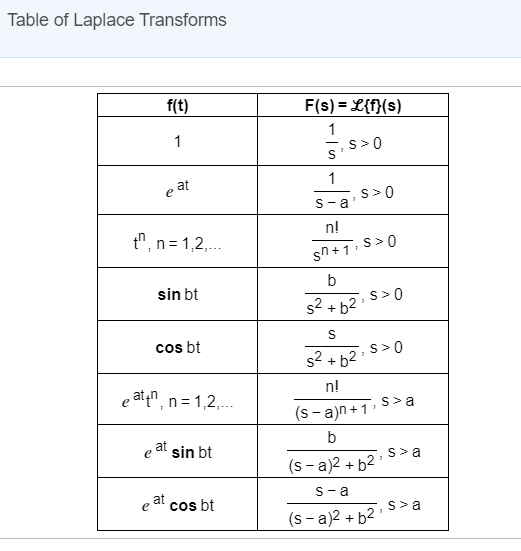
Solved Determine The Inverse Laplace Transform Of The Chegg Com

Inverse Laplace Transform Formula And Simple Examples Wira Electrical